Roice's G+ Posts

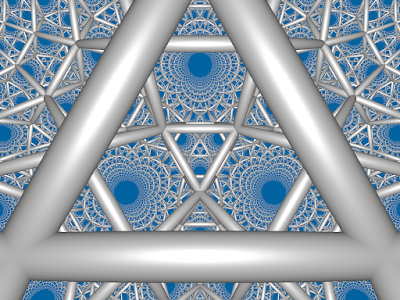
Rectified {4,4,4} The regular {4,4,4} has square tiling cells.  The vertex figure is a square tiling too. https://en.wikipedia.org/wiki/Order-4_square_tiling_honeycomb Therefore, the rectified {4,4,4} contains rectified square tiling cells and square tiling cells.  We saw earlier that a rectified square tiling is just another square tiling, so the rectified {4,4,4} has square tiling cells and square tiling cells.  Wait a minute, that's just one kind of cell...and hmmmm...that cell is regular.  This doesn't sound like a uniform honeycomb. And indeed it isn't.  The rectified {4,4,4} is the regular {4,4,3}!  This came as a surprise to me when I saw the rendering.  It's the only honeycomb to do this after rectification, so I thought it would be a good one to end on.   While there are 15 regular honeycombs in hyperbolic space, rectification produces just 14 new honeycombs. I hope you enjoyed having these pictures trickle out one-a-day.  I'll likely jump back into less f

Rectified {4,3,6} Ideal cubical cells make up the regular {4,3,6}.  6 cells meet at every edge, and an infinite number meet at every vertex, in the pattern of a triangular tiling. https://en.wikipedia.org/wiki/Order-6_cubic_honeycomb The rectified {4,3,6} has cuboctahedral and triangular tiling cells.  All the cuboctahedra connect to each other through their square faces, and connect to triangular tilings through their triangle faces. It's cool to compare this honeycomb with the rectified {3,4,4}.  That also has cuboctahedral cells, but the connections switch.  Cuboctahedra touch through triangle faces and connect to the Euclidean tiling cells via their square faces. https://plus.google.com/112844794913554774416/posts/WC8uFvXpPEY Last post on rectified honeycombs tomorrow!

Rectified {6,3,5} The regular {6,3,5} is one of four regular honeycombs composed of hexagonal tiling cells.  Since 5 cells surround each edge, it is also called the "order-5 hexagonal tiling honeycomb". http://en.wikipedia.org/wiki/Order-5_hexagonal_tiling_honeycomb The rectified {6,3,5} has trihexagonal tiling and icosahedral cells.  The vertex figure is a pentagonal prism. It's nice to look at this honeycomb in the context of rectifications of {6,3,3}, {6,3,4}, and {6,3,6}.  We've seen all those, so I'll include links to them here for reference.   What's the same between all four honeycombs in the rectified {6,3,r} progression?  What's different? Rectified {6,3,3} https://plus.google.com/112844794913554774416/posts/CC2uv2atXPW Rectified {6,3,4} https://plus.google.com/112844794913554774416/posts/9ssK7Mzejcj Rectified {6,3,6} https://plus.google.com/112844794913554774416/posts/2EBs3KT4UVo

I really enjoyed watching Henry's recent talk on 4D sculptures this evening! Originally shared by Henry Segerman I gave the 2014 Hari Shankar Memorial lecture at the University of Northern Iowa, on "How to make sculptures of 4-dimensional things". Featuring work with Saul Schleimer, Vi Hart and Will Segerman.  http://www.youtube.com/watch?v=NT0u-EINURc

Rectified {3,4,4} Like the {5,3,6}, the regular {3,4,4} is another honeycomb with ideal cells, but this time the cells are octahedra.  4 cells meet at every edge, and an infinite number meet at every vertex (in the pattern of a square tiling). http://en.wikipedia.org/wiki/Order-4_octahedral_honeycomb The rectified {3,4,4} has cuboctahedral and square tiling cells.  The edge lengths are finite. http://en.wikipedia.org/wiki/Cuboctahedron http://en.wikipedia.org/wiki/Square_tiling We're almost done going through these.  Only 3 more rectified honeycombs to visit, with at least one surprise in store, so stay tuned :) Of course, there are a huge number of additional uniform honeycombs to be rendered.  I ordered a book today which I hope will help me be able to approach more of them. Reflection Groups and Coxeter Groups by James Humphreys http://www.amazon.com/gp/product/0521436133/?tag=gravit-20

Rectified {5,3,6} The dodecahedron just might be the most beautiful platonic solid.  Even if you disagree, regular honeycombs of the form {5,3,r} are certainly engaging because they are built of dodecahedra.  When r is 6, the dodecahedral cells become ideal .  Their vertices have gone to infinity. https://en.wikipedia.org/wiki/Order-6_dodecahedral_honeycomb The rectified {5,3,6} has icosidodecahedral and triangular tiling cells.  Before peeking at the picture, I wondered if the icosidodecahedral cells might also be ideal.  But again rectification makes the infinite edge lengths of the regular honeycomb finite, and the cells are no longer ideal. But I still wonder... do you think there exists a uniform honeycomb that does have ideal icosidodecahedral cells?  My guess is yes, and I bet wendy krieger knows!

Rectified {6,3,6} Like the {3,6,3}, the geometry of the regular {6,3,6} can be quite strange because of all the infinities.   https://en.wikipedia.org/wiki/Order-6_hexagonal_tiling_honeycomb Thank goodness for the Poincaré ball model, which allows interaction with all the elements in a finite way.  The cells of the rectified {6,3,6} are trihexagonal tilings and triangular tilings.  The vertex figure is a hexagonal prism.

Check out this extremely cool art project shared with me by my brother Robert Nelson.  The honeycombs I've been posting are generated similarly.   It's hard to believe, but 4 mirrors suffice to bring about all the complexity I've been showing (the 4 faces of a tetrahedron).   For regular honeycombs, you can also use the faces of a cell as generating mirrors.  In this case, the 6 faces of a cube are producing the {4,3,4} Euclidean honeycomb. I like that this art installation bends some of the mirrors (both outward and inward), bringing us into the worlds of spherical and hyperbolic geometry.   I wonder if that was part of their motivation. Be sure to watch the video! http://themindunleashed.org/2014/03/happens-build-cube-one-way-mirrors.html http://themindunleashed.org/2014/03/happens-build-cube-one-way-mirrors.html

Rectified {3,6,3} The regular {3,6,3} honeycomb has triangular tiling cells. The vertex figure is a hexagonal tiling.   http://en.wikipedia.org/wiki/Triangular_tiling_honeycomb It is one of three especially exotic regular honeycombs because infinities exist in both its cells and vertex figure.   - Each cell has an infinite number of facets. - Every cell facet is infinite in extent.   - All the vertices live at infinity.   - An infinite number of cells meet at every vertex.  - All edges are infinitely long.   - The in-radius, mid-radius, and circum-radius are all infinite.   - The volume of each cell is infinite.   - Each cell of the honeycomb is inscribed by the entirety of hyperbolic space, which makes the cells somehow feel "bigger" than those in many of the other honeycombs. In short, just about every property of this thing has flown the coop. But check out the rectified {3,6,3} in these images.  Rectification produces finite edge lengths again!  How'd that happen? Ca

Love this!  I've seen "little planet" pictures before, but not video. Robert Nelson, check out the following article, Mathematics Meets Photography , for some of the cool math behind these images (in particular stereographic projection). http://www.maa.org/publications/periodicals/math-horizons/mathematics-meets-photography A more in-depth version of the article is in the book The Best Writing on Mathematics 2012 . http://www.amazon.com/The-Best-Writing-Mathematics-2012/dp/0691156557/?tag=gravit-20 Originally shared by Robert Nelson Little worlds all around us - Video captured from 360 degree spherical panorama

Rectified {6,3,4} John Baez made a post this morning about the regular {6,3,4}, so let's look at the rectified {6,3,4} for today.  I love reading other's descriptions because it helps me look at things differently than I do on my own.  Here is John's post: https://plus.google.com/117663015413546257905/posts/BUGkT3k5GKD Like the {6,3,3} from yesterday, this is another honeycomb with hexagonal tilings for cells, but now the vertex figure is an octahedron.   The rectification is also similar because we get trihexagonal tiling cells again.  The main difference is that those cells are interspersed with octahedra instead of tetrahedra. The vertex figure of the rectified {6,3,4} is a square prism (a cuboid).

Rectified {6,3,3} The regular {6,3,3} is a honeycomb with hexagonal tilings as cells. The vertex figure is a tetrahedron. http://en.wikipedia.org/wiki/Hexagonal_tiling_honeycomb When we rectify the {6,3,3}, the hexagonal tilings turn into a uniform tiling with triangles and hexagons called the trihexagonal tiling . http://en.wikipedia.org/wiki/Trihexagonal_tiling And we get tetrahedral cells where the vertices used to live.   When I look at these images, my mind tends to see an intricate lattice of tetrahedra connected up at their tips.  The vertices are all identical, which is a property of uniform honeycombs (they are vertex transitive ).  Take a look at a vertex of the rectified {6,3,3} and see if you can deduce the shape of the vertex figure.  I'll put the answer in the comments tomorrow.

Rectified {4,4,3} I've posted images of 5 rectified hyperbolic honeycombs so far, but there are 15 total, one for each regular honeycomb.  I finished up images of the complete set this weekend, but thought it'd be nice to go through the remaining one-a-day (in random order), so they can get some individual attention.  If you want to jump straight to seeing all of them, the full set is on my wiki user page:  https://commons.wikimedia.org/wiki/User:Roice3 The regular {4,4,3} honeycomb has {4,4} cells and a {4,3} vertex figure.  {4,4} denotes a Euclidean square tiling.  The {4,3} is a cube.   Therefore, the rectified {4,4,3} has rectified {4,4} cells and cubical cells.  A rectified {4,4} tiling is interesting because it is just another {4,4} tiling.  Two properties lead to this.  First, a rectified square is just another smaller square.  Second, the vertex figure of a {4,4} is a square. See if you can find the two cell types in the images: cubes and square tilings.  Unlike many of

Experimenting with Different Projections No new honeycomb here, but some new and strange views.  Which do you like best? This is the rectified {3,3,6}.  In all these images the camera is at the origin of the Poincare ball model and looking in the same direction.  Only the projection from the 3D model to the screen is changing.  Some of the results get pretty wacky, and details on the various projections are here: http://wiki.povray.org/content/Reference:Camera#Types_of_Projection Tom Ruen, I think the closest to what you were asking about last night is the "Ultra-wide 180 degrees".  Maybe this would be the best projection for wikipedia images?

Rectified {3,3,6} through a Fisheye Lens Here's one more rectified honeycomb for this evening.  The {3,3,6} has ideal tetrahedra for cells, and the vertex figure is a {3,6} tiling.  So the rectified form has rectified tetrahedra (octahedra) and {3,6} tilings for cells. This is the first I've tried the fisheye view, and I really like it.  You can see much more of the honeycomb, and this makes me want to return to some of the previous renderings and try them anew.

More fun with honeycombs in hyperbolic 3-space... Rectification Check out the four compact regular H³ honeycombs rectified .  Rectification is a special case of truncation , where you truncate all the way to the midpoints of edges.   Regular H³ honeycombs contain one type of polyhedron, but rectifying them yields a new honeycomb composed of two different types of polyhedra.  One is the original polyhedron rectified, and the other is the vertex figure of the parent honeycomb.   Because rectified honeycombs have two cell types, they are not regular, but they still have a great deal of symmetry and are also called uniform or Archimedean honeycombs. Let's see what two polyhedra live in a rectified {5,3,4}, the cover image of this post.  The parent honeycomb is built from dodecahedra, and the the vertex figure is an octahedron.  So the rectified honeycomb contains rectified dodecahedra (a.k.a. icosidodecahedra) and octahedra, all fitting neatly together. (aside: you can read off