Honeycombs!
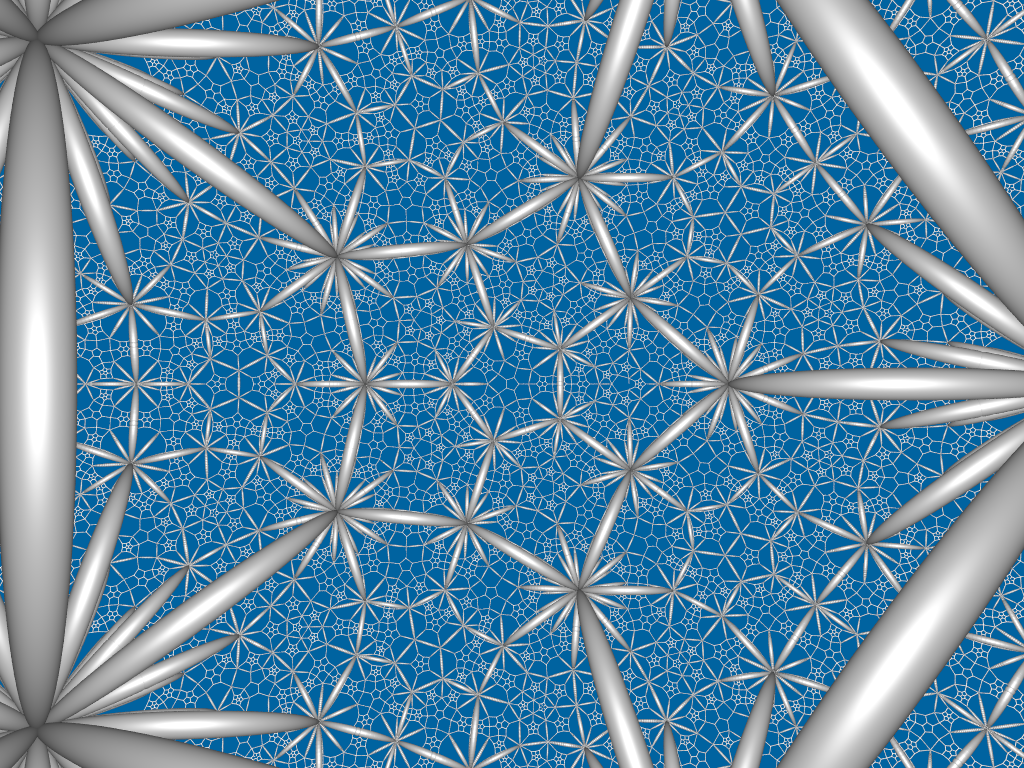
Honeycombs! Here is a complete set of images of all 15 regular honeycombs in hyperbolic 3-space. Thanks to Tom Ruen for encouraging me to make these for wikipedia. A honeycomb is when you take a bunch of polyhedra and pack them together with no gaps. The polyhedra in a honeycomb are called "cells". The background colors group the honeycombs as follows: Teal: Cells are finite Blue: Cells have "ideal" vertices (vertices that live at infinity) Green: Cells have an infinite number of facets Cyan: Cells have ideal vertices and an infinite number of facets All the images show the honeycombs in the Poincare Ball model, with the camera placed either at the origin or on the boundary of the ball. They were generated with custom C# code and rendered with POV-Ray. Links for further study "Regular Honeycombs in Hyperbolic Space", Coxeter: http://www.mathunion.org/ICM/ICM1954.3/Main/icm1954.3.0155.0169.ocr.pdf YouTube video by Henry Segerman explaining a few of