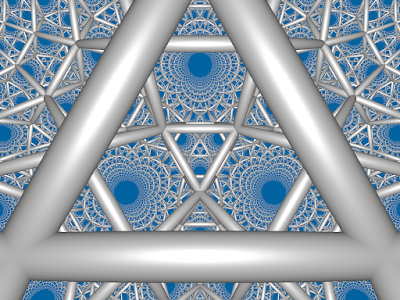
Rectified {3,4,4}
Rectified {3,4,4}
Like the {5,3,6}, the regular {3,4,4} is another honeycomb with ideal cells, but this time the cells are octahedra. 4 cells meet at every edge, and an infinite number meet at every vertex (in the pattern of a square tiling).
http://en.wikipedia.org/wiki/Order-4_octahedral_honeycomb
The rectified {3,4,4} has cuboctahedral and square tiling cells. The edge lengths are finite.
http://en.wikipedia.org/wiki/Cuboctahedron
http://en.wikipedia.org/wiki/Square_tiling
We're almost done going through these. Only 3 more rectified honeycombs to visit, with at least one surprise in store, so stay tuned :)
Of course, there are a huge number of additional uniform honeycombs to be rendered. I ordered a book today which I hope will help me be able to approach more of them.
Reflection Groups and Coxeter Groups by James Humphreys
http://www.amazon.com/gp/product/0521436133/?tag=gravit-20




Let me count the ways. There are five groups of finite extent, 534, 353, 535, 53A, 3334:, 3335:, 3434:, 3435:, and 3535: , which make by uniforms, 15, 9, 9, 4, 9, 9, 5, 9, and 5. To this are known non wythoffian ones, L438, 1, of 353, 2, of the pyritohedral, an infinite class, and two more, of L84A, 1, of the snub verf, 6, i can't think of any more here.
ReplyDeleteOf the finite extent, there are various wythoffian groups, 17 belonging to the crystalographic, two to 536, and four sundries. There are a number of non wythoffian groups as well, though i have not looked at all these yet.
There is for example, a chiral tiling of dodecahedra and pentagonal antiprisms, there is a separate borrochemean for every polyoon except the square It exists for the square too, as 4,3,5. There's three for every snub, and so many more to meet.
ReplyDeleteI really like Humphreys' book on Coxeter groups. When a new PhD student starts with me, that's usually the first thing they read.
ReplyDeleteI had a flick through of the book. I put an order in for it, but it looks a lot like the roman numbers we staved off with the new processes.
ReplyDeleteI got my copy of humphreys today. Why do they make it so hard? I mean, they don't even touch on hyperbolic frieze groups. Coxeter was more interested in groups. I spent some time talking with one of his phd's (Norman Johnson), who seemed to have issues when you put irrational numbers into the schlafli sympol, kind of like {3.14159265359}. I mean, how would he deal with the hyperbolic polygons as vertex figures. Like {4, W6.82842}. The stuff in humphries does not cover even the finite content groups, like the lamina-apiculates, or the fancy crossed frieze groups of 8,3,4., or 8,4,A. Still, the Art unifies the geomrties in a startlingly interesting way.
ReplyDeleteI suspect you have to go to uni rather than cutting your own way there. Maybe i really should put the Art out there, if i find a publisher.
Here's a link to the magic that Roice will need
ReplyDeletehttps://plus.google.com/113926680576216305183/posts/2SgH2eoSwGT
On the other hand, my method is quite at home when all of the vertices are ideal, and even quite at home with the likes of {4,5,4} and {8,3,4}.
ReplyDelete