{7,3} Surfboard



{7,3} Surfboard

For our tenth anniversary, Sarah Nemec-Nelson planned a perfect gift, a custom surfboard that I could decorate with mathematical art.  We've spent a lot of time in Galveston the last few years, and I've really enjoyed learning to surf.

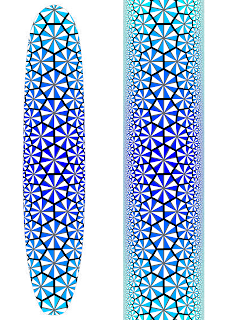
I've been tuning a number of ideas, finally settling on this.  The surfboard deck will look like the left portion of the image.  The pattern used is on the right.  It is the {7,3} hyperbolic tiling in Vladimir Bulatov's band model, along with a gray outline of its {3,7} dual and a colorful Coxeter complex.  Like the Poincaré disk and upper half plane, the band model is a conformal model of the hyperbolic plane.  The coloring is based on ideas developed with Henry Segerman for our paper Visualizing Hyperbolic Honeycombs.

The surfboard will be shaped by James Fulbright of Strictly Hardcore Surf Specialties, and BoardLams will do the printing (on fiberglass).  I'm stoked!  I'll be sure to post pictures of the end result.

Relevant Links

Vladimir's band model
bulatov.org/math/1001

More on the band model, by Jos Leys (in French, but google translate helps)
images.math.cnrs.fr/Ringworld.html

Visualizing Hyperbolic Honeycombs
arxiv.org/abs/1511.02851

Strictly Hardcore Surf Specialties
www.surfspecialties.com

BoardLams
www.boardlams.com

Comments

Post a Comment

Popular posts from this blog

Hyperbolic Hopf Fibrations

Image
Hyperbolic Hopf Fibrations The Hopf Fibration of S^3 is amazing and beautiful.  Rather than describe it here, I'll point you to a lovely online reference with pictures and videos by Niles Johnson. nilesjohnson.net/hopf.html To understand it better (and fibrations in general), I recommend this talk by Niles too. www.youtube.com/watch?v=QXDQsmL-8Us It turns out there is an analogue of the Hopf fibration for H^3.  In fact, there is not just one "fiberwise homogenous" fibration in the hyperbolic case.  There is a 2-parameter family of them, plus one additional fibration that does not fit the family.  As with S^3, fibers in the H^3 cases are geodesics.  They are ultraparallel in fibrations from the family, and parallel in the exceptional fibration.  I found the following dissertation by Haggai Nuchi a good intro and resource to help think about all this. www.math.upenn.edu/grad/dissertations/NuchiThesis.pdf I'm posting three early pictures of H^3 fibrations belo...
Read more

76 Unique Honeycombs

76 Unique Honeycombs Last weekend, Tom Ruen and I hit the milestone of uploading to wikipedia at least one image for 9 families of compact, Wythoffian, uniform H3 honeycombs, a total of 76 unique honeycombs. http://en.wikipedia.org/wiki/Uniform_honeycombs_in_hyperbolic_space You can easily browse all the images on my wiki user page: http://commons.wikimedia.org/wiki/User:Roice3 - Compact means the cells are finite in extent.  - Wythoffian means we can generate them using a kaleidoscopic construction, that is by reflecting in mirrors.    - Uniform means they are vertex transitive and have uniform polyhedral cells. There may even be more honeycombs that meet all these criteria, I don't know. ( update: see Tom's comment below! )  I do know there are hundreds more which don't meet one or more of these criteria, many undiscovered.  In fact, there are infinitely more because there are some infinite families of honeycombs.  wendy krieger continues to discover and enumerate more...
Read more