Nonagonal Firepit
Nonagonal Firepit
For our 9th anniversary, Sarah Nemec-Nelson and I decided to gift each other a custom firepit with 9 sides. Through her interior design business, Sarah has an excellent contact named Brent Clifton who was excited about the project. He has a computer controlled plasma cutter, and the result is amazing. It's so heavy duty it will likely outlast our short time on this planet.
There are two nonagons in the design, the base and the rim. During design, Brent's wife had a very nice suggestion - scale the rim to the base by the golden ratio φ. But this would be more appropriate for a pentagonal firepit, since φ is the ratio of a pentagon's diagonal to its edge length. The nonagon has its own set of ratios, three of them in fact. Here is a paper all about it!
Peter Steinbach, Golden fields: a case for the heptagon, Mathematics Magazine 70 (Feb., 1997), 22-31. Available at http://www.jstor.org/stable/2691048
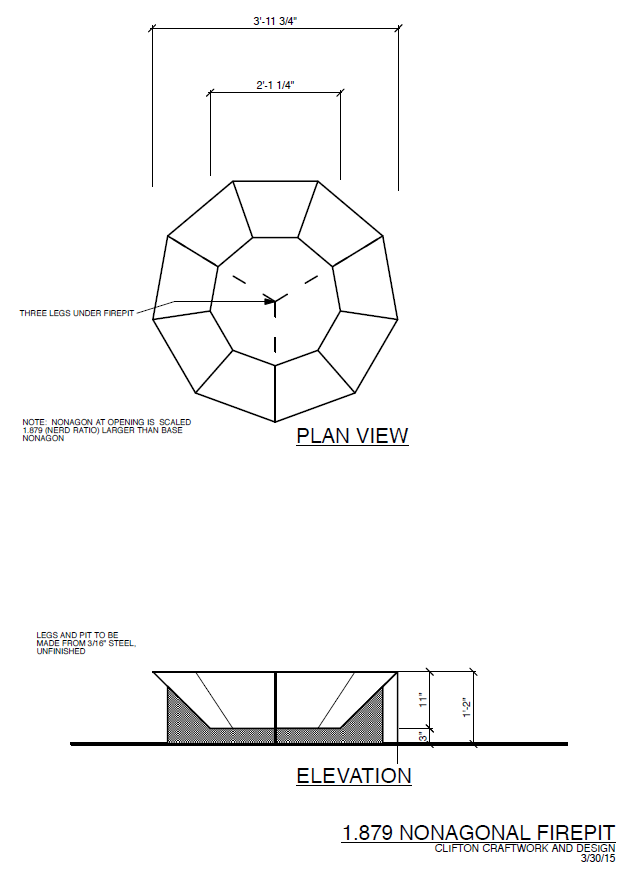
So we chose to use the smallest of the three nonagon ratios, which is equal to sin(2*pi/9)/sin(pi/9) ~ 1.879. Brent officially dubbed this the "nerd ratio". In addition to scaling by the nerd ratio, one can also construct the smaller nonagon of the base by drawing the second longest diagonals into the larger nonagon of the rim. They intersect to produce the smaller nonagon (see the second image below).
Another design decision was the handles. Since there are an odd number of sides, we couldn't easily place two handles on opposite sides - one of them would require a unique shape. However, using three handles turned out great for the nonagon. This wouldn't have worked as well for our 7th anniversary.
I've been meaning to post this for some time (Sarah and I are on the cusp of celebrating 10 years), but better late than never. We've already had many pleasant evenings by the fire.
Relevant Links
Nonagon (aka Enneagon)
https://en.wikipedia.org/wiki/Nonagon
Golden Fields: A Case for the Heptagon
The paper above was previously available online without a paywall, but the link doesn't seem to be working at the moment, timing out. In case it returns, here it is:
http://sylvester.math.nthu.edu.tw/d2/imo-training-geometry/reg-poly.pdf
Golden Fields, Generalized Fibonacci Sequences, and Chaotic Matrices
Another useful paper about these generalized ratios. This one has nice formulas and explicitly lists the decimal ratio we used.
http://www.scipress.org/journals/forma/pdf/1904/19040367.pdf
Clifton Craftwork and Design
Highly recommended if you are in the Austin area and want to do a custom metal project! Brent was enthusiastic, accommodating, and hilarious while working on our project.
www.cliftoncraftwork.com
Maison Interior Design
I'm biased of course, but Sarah really is an amazing designer!
www.maisonaustin.com




For our 9th anniversary, Sarah Nemec-Nelson and I decided to gift each other a custom firepit with 9 sides. Through her interior design business, Sarah has an excellent contact named Brent Clifton who was excited about the project. He has a computer controlled plasma cutter, and the result is amazing. It's so heavy duty it will likely outlast our short time on this planet.
There are two nonagons in the design, the base and the rim. During design, Brent's wife had a very nice suggestion - scale the rim to the base by the golden ratio φ. But this would be more appropriate for a pentagonal firepit, since φ is the ratio of a pentagon's diagonal to its edge length. The nonagon has its own set of ratios, three of them in fact. Here is a paper all about it!
Peter Steinbach, Golden fields: a case for the heptagon, Mathematics Magazine 70 (Feb., 1997), 22-31. Available at http://www.jstor.org/stable/2691048
So we chose to use the smallest of the three nonagon ratios, which is equal to sin(2*pi/9)/sin(pi/9) ~ 1.879. Brent officially dubbed this the "nerd ratio". In addition to scaling by the nerd ratio, one can also construct the smaller nonagon of the base by drawing the second longest diagonals into the larger nonagon of the rim. They intersect to produce the smaller nonagon (see the second image below).
Another design decision was the handles. Since there are an odd number of sides, we couldn't easily place two handles on opposite sides - one of them would require a unique shape. However, using three handles turned out great for the nonagon. This wouldn't have worked as well for our 7th anniversary.
I've been meaning to post this for some time (Sarah and I are on the cusp of celebrating 10 years), but better late than never. We've already had many pleasant evenings by the fire.
Relevant Links
Nonagon (aka Enneagon)
https://en.wikipedia.org/wiki/Nonagon
Golden Fields: A Case for the Heptagon
The paper above was previously available online without a paywall, but the link doesn't seem to be working at the moment, timing out. In case it returns, here it is:
http://sylvester.math.nthu.edu.tw/d2/imo-training-geometry/reg-poly.pdf
Golden Fields, Generalized Fibonacci Sequences, and Chaotic Matrices
Another useful paper about these generalized ratios. This one has nice formulas and explicitly lists the decimal ratio we used.
http://www.scipress.org/journals/forma/pdf/1904/19040367.pdf
Clifton Craftwork and Design
Highly recommended if you are in the Austin area and want to do a custom metal project! Brent was enthusiastic, accommodating, and hilarious while working on our project.
www.cliftoncraftwork.com
Maison Interior Design
I'm biased of course, but Sarah really is an amazing designer!
www.maisonaustin.com







Reminds me of a solar cooker.
ReplyDeleteа мне немного напомнило музыкальный инструмент Ханг
ReplyDelete