A sunflower at infinity

Originally shared by John Baez

A sunflower at infinity

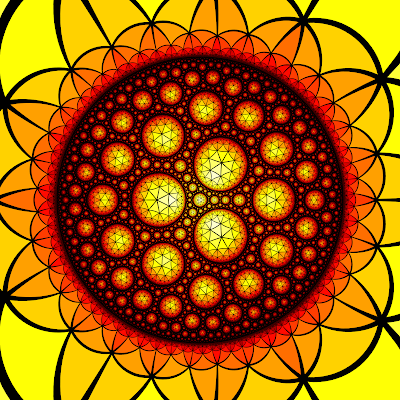
This picture by Roice Nelson shows the 'view at infinity' of a honeycomb in hyperbolic space.

A honeycomb is a way of chopping space into polyhedra.  For example, we can chop ordinary 3d space into cubes.  This is called the {4,3,4} honeycomb.  Why?

• a square has 4 sides so its symbol is {4}

• a cube has 3 squares meeting at each corner so its symbol is {4,3}

• the cubical honeycomb has 4 cubes meeting at each edge so its symbol is {4,3,4}

The picture here is a view of the {3,3,7} honeycomb.  This is defined in the same sort of way, but it doesn't fit into ordinary Euclidean space.  It fits into a curved space called hyperbolic space!   The honeycomb extends forever, and it forms this pattern where it meets the 'plane at infinity' of hyperbolic space.

For links to related pictures, visit my American Mathematical Society blog Visual Insight:

http://blogs.ams.org/visualinsight/2014/09/01/intersection-of-337-honeycomb-and-the-plane-at-infinity/

#geometry