Roice's G+ Posts

Closing out the series with a parabolic transformation. The horosphere center is at infinity, so this "limit rotation" looks like a translation in the upper half space model. I've made a page that puts the entire set of animated gifs along with explanations in one place. I hope it can help provide an intuition about symmetries of hyperbolic 3-space! http://roice3.org/h3/isometries/

Amour et maths! My first image to make a book cover is on the most recent French edition of Love and Math by Edward Frenkel :D Can you figure out the three angles of the triangles in the tiling? https://editions.flammarion.com/Catalogue/champs-sciences/amour-et-maths

Let's look at the previous one with the fixed points moved to the origin and infinity. Now it appears like we are scaling Euclidean space, but the transformation depicted preserves distances in hyperbolic space! Just one more image tomorrow to finish this series.

The last class of symmetries to share are hyperbolic transformations. Points emanate from a source and disappear into a sink. A cylinder translates along its axis in hyperbolic space, while the boundary preserves a family of ideal circles sharing the 2 fixed points.

Incredible Originally shared by Henry Segerman The "Making of" video Vi Hart and I made for our space-time symmetry video, "Peace for Triple Piano". https://youtu.be/x1zJoU6Luss

An elliptic with the fixed geodesic a vertical line through the origin. Less dramatic visuals, but still interesting. This is the first of the set to simultaneously show a length preserving transform both of Euclidean space and of hyperbolic space in the upper half space model.

Next up: elliptic transformations These are analogous to Euclidean rotations. The motion fixes an entire line in hyperbolic space, the core geodesic of the banana shape. Points traverse parallel circles on the banana, which is a cylinder in hyperbolic space. For this animation, it would also have been natural to pick a geodesic plane orthogonal to the banana as a surface to render. Such planes are filled with concentric circles, culminating in an ideal circle (a member of the family of concentric circles we see here on the boundary). Something to add to the shader. http://bit.ly/2BNdwS4

Conjugate loxodromic isometry Another loxodromic length-preserving transformation of hyperbolic 3-space, conjugate to the one from yesterday. The surface we saw above the boundary plane is now a cone (in the upper half space model - it's a cylinder surrounding a geodesic in hyperbolic space). The motion still fixes 2 ideal points, now at the origin and infinity, and we see logarithmic spirals on the boundary. Click F in the shader to see this view: http://bit.ly/2BNdwS4

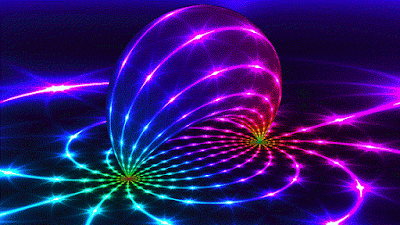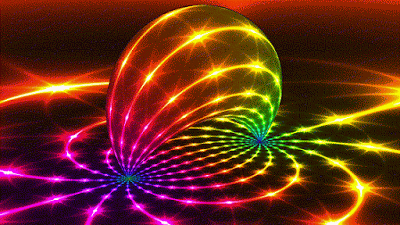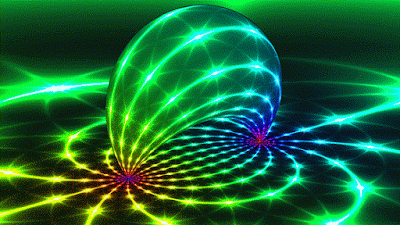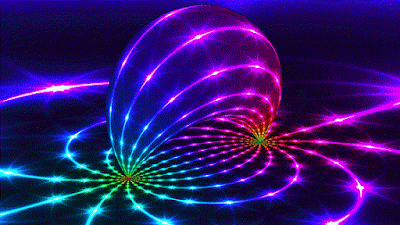
Isometries of hyperbolic 3-space Here is the first of a set of animated gifs showing basic isometries of hyperbolic 3-space. This one displays a loxodromic transformation. I'll post one gif a day for a bit, but the shader rendering them all is ready for your tinkering! https://www.shadertoy.com/view/MstcWr Update based on comments I should have said a little about the banana shape. It is explained some in the shader comments, which I'll copy here. Also, see the comment thread below! This shader shows basic isometries (length preserving transformations) of hyperbolic 3-space in the upper half space model. The z=0 plane is the boundary plane-at-infinity. There are four classes of transformations: parabolic, elliptic, hyperbolic, and loxodromic. These may fix 1 or 2 ideal points on the boundary plane. In general, any Mobius transformation applied to the boundary plane will extend to an isometry of hyperbolic 3-space, but all can be built by composition of the basic transformati

Discoball! ...or a shader that demonstrates a parabolic transformation in the upper half space model of hyperbolic 3-space. The flat plane is the "plane at infinity". The sphere is a horosphere that kisses the plane at the origin, which is also the ideal center of the horosphere and the single fixed point of the transformation. Interact with and edit the shader here: http://bit.ly/2Cd8Cuq I hope to extend this shader to allow displaying other classes of transformations of hyperbolic space (elliptic, hyperbolic, loxodromic). This was my first go at using Shadertoy. It's pretty cool and relatively easy to use, especially because there is such a large library of examples. I recommend the tutorial at the following link to get started. http://jamie-wong.com/2016/07/15/ray-marching-signed-distance-functions/ https://youtu.be/ZKL9lGX9XHc

A ghostly 2-skeleton I continue to play with sections of honeycombs, most recently sections of thickened 2-skeletons. So many avenues for exploration! For this picture, I took 500 frames from a video experiment, made each slightly translucent, and stacked them back-to-front. The animated frames are here: https://youtu.be/7c9ZAGcK6F0 Even though the sections were taken from the 2-skeleton, the 1-skeleton of the honeycomb jumps out. The image is a nice verification that the sections are correct. I'll leave it as an exercise to identify this particular honeycomb. Hint: it has two cell types.

A spherical section of a honeycomb of buckyballs, with the sphere flying through hyperbolic space! https://tinyurl.com/y8hdw6lm