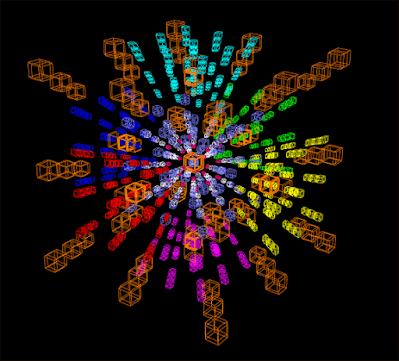
Originally shared by John Baez This the {6,3,3} honeycomb , as drawn by Roice Nelson. A 3-dimensional honeycomb is a way of filling 3d space with polyhedra. It’s the 3d analogue of a tiling of the plane. But besides honeycombs in ordinary Euclidean space, we can also have them in hyperbolic space . This is a curved 3d space. The {6,3,3} honeycomb lives in hyperbolic space. That's why it looks weirdly distorted. Actually all the hexagons are the same size... but we have to warp hyperbolic space to draw it in ordinary space. You can learn more about all these concepts by going to my new blog, Visual Insight : http://blogs.ams.org/visualinsight/2013/09/15/633-honeycomb-in-upper-half-space/ But let me just answer one obvious question: why is it called the {6,3,3} honeycomb? {6,3,3} is a Schläfli symbol. The symbol for the hexagon is {6}. The symbol for the hexagonal tiling of the plane is {6,3} because 3 hexagons meet at each vertex. S...